Problem: Clone Graph

​leetcode.com/problems/clone-graph/submissions/

Given a reference of a node in a connected undirected graph.

Return a deep copy (clone) of the graph.

Each node in the graph contains a val (int) and a list (List[Node]) of its neighbors.

class Node {
    public int val;
    public List<Node> neighbors;
}

 

Test case format:

For simplicity sake, each node's value is the same as the node's index (1-indexed). For example, the first node with val = 1, the second node with val = 2, and so on. The graph is represented in the test case using an adjacency list.

Adjacency list is a collection of unordered lists used to represent a finite graph. Each list describes the set of neighbors of a node in the graph.

The given node will always be the first node with val = 1. You must return the copy of the given node as a reference to the cloned graph.

 

Example 1:

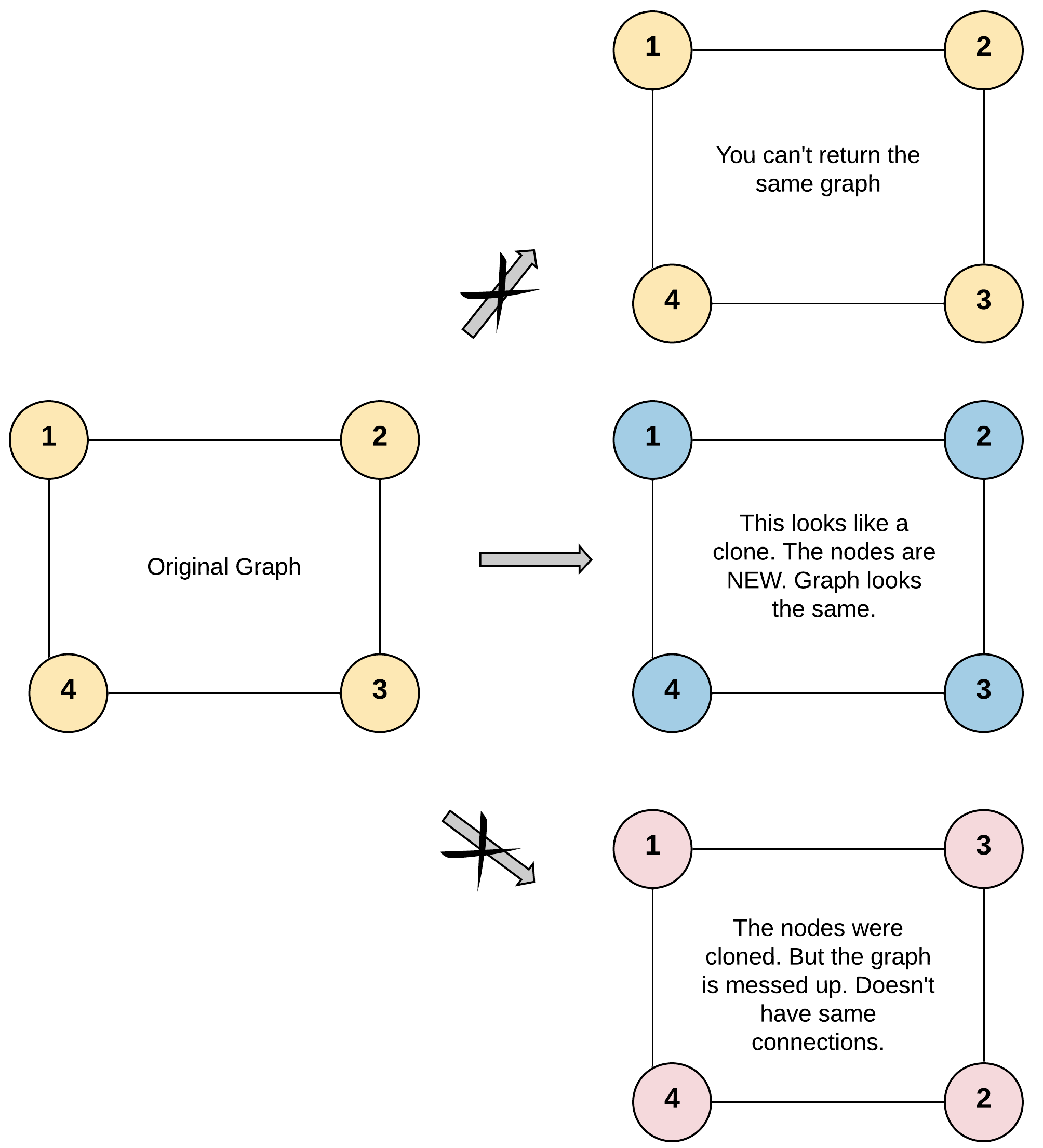
Input: adjList = [[2,4],[1,3],[2,4],[1,3]]
Output: [[2,4],[1,3],[2,4],[1,3]]
Explanation: There are 4 nodes in the graph.
1st node (val = 1)'s neighbors are 2nd node (val = 2) and 4th node (val = 4).
2nd node (val = 2)'s neighbors are 1st node (val = 1) and 3rd node (val = 3).
3rd node (val = 3)'s neighbors are 2nd node (val = 2) and 4th node (val = 4).
4th node (val = 4)'s neighbors are 1st node (val = 1) and 3rd node (val = 3).

Example 2:

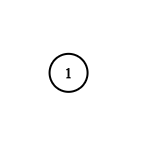
Input: adjList = [[]]
Output: [[]]
Explanation: Note that the input contains one empty list. The graph consists of only one node with val = 1 and it does not have any neighbors.

Example 3:

Input: adjList = []
Output: []
Explanation: This an empty graph, it does not have any nodes.

Example 4:

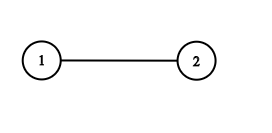
Input: adjList = [[2],[1]]
Output: [[2],[1]]

 

Constraints:

• 1 <= Node.val <= 100
• Node.val is unique for each node.
• Number of Nodes will not exceed 100.
• There is no repeated edges and no self-loops in the graph.
• The Graph is connected and all nodes can be visited starting from the given node.

SOLUTION:
​DFS Graph traversal is similar to tree traversal, except due to cycles, we need to keep the list of visited nodes and backtrack if we hit a visited node

Because in the scenario below, we have to backtrack from a clone, just a list of visited nodes won't do, so we keep a dictionary that maps the visited node in the original graph to the node in the clone graph

"""
# Definition for a Node.
class Node:
    def __init__(self, val = 0, neighbors = None):
        self.val = val
        self.neighbors = neighbors if neighbors is not None else []
"""
            
class Solution:
    def __init__(self):
        self.visited = {}
        
    def cloneGraph(self, node: 'Node') -> 'Node':
        if not node:
            return node
        
        if node in self.visited:
            return self.visited[node]
        
        clone = Node(node.val, [])
        
        self.visited[node] = clone
        
        if node.neighbors:
            for n in node.neighbors:
                clone.neighbors.append(self.cloneGraph(n))
        
        return clone

​Word Break

https://leetcode.com/problems/word-break/​

Given a non-empty string s and a dictionary wordDict containing a list of non-empty words, determine if s can be segmented into a space-separated sequence of one or more dictionary words.

Note:

• The same word in the dictionary may be reused multiple times in the segmentation.
• You may assume the dictionary does not contain duplicate words.

Example 1:

Input: s = "leetcode", wordDict = ["leet", "code"]
Output: true
Explanation: Return true because "leetcode" can be segmented as "leet code".

Example 2:

Input: s = "applepenapple", wordDict = ["apple", "pen"]
Output: true
Explanation: Return true because "applepenapple" can be segmented as "apple pen apple".
             Note that you are allowed to reuse a dictionary word.

Example 3:

Input: s = "catsandog", wordDict = ["cats", "dog", "sand", "and", "cat"]
Output: false

def findWordBreak(s, wordDict, seen):
    
    if not s:
        return True
    
    if s in seen:
        return seen[s]
    
    i=0
    ret = False
    
    while not ret and i < len(wordDict):
        if  s.startswith(wordDict[i]):
            ret = findWordBreak(s[len(wordDict[i]):], wordDict, seen)
        i+=1
        seen[s] = ret
    
    return ret
    
class Solution:
    def wordBreak(self, s: str, wordDict: List[str]) -> bool:
        return findWordBreak(s, wordDict, {})